2 a I The product of uncertainty between energy and time is
A) ≥h/2p B) ≥h/4p C) h/2p D)≤h/4p
II According to Max Born approximation, y2 represents
A) Energy density B) Particle density C) Probability density D) Charge density
III The first permitted energy level is called
A) Excited energy level B) Zero point energy C) Ground level D) None of these
IV) The wavefunction associated with a material particle is
A) Finite B) Continuous C) Single valued D) All the above
b. Set up time independent one dimensional Schrödinger wave equation.
c. What are eigen values and eigen function
d. Compute first three permitted energy values for an electron in a box of width 4Å.
(4+6+6+4)
Dec08/Jan09
2 a 1) The product of uncertainty between angular momentum and angular displacement is
A) ≥h/2p B) ≥h/4p C) h/2p D)≤h/4p
2) Kinetic energy of electron acclerated by a voltage 50 Volts
A) 50 eV B) 10 eV C) 5 eV D) 15 eV
3) The energy of the lowest state in one dimensional potential box of length ‘a’ is
A) Zero B) 2h2/8ma2 C) h2/8ma2 D) h/8ma2
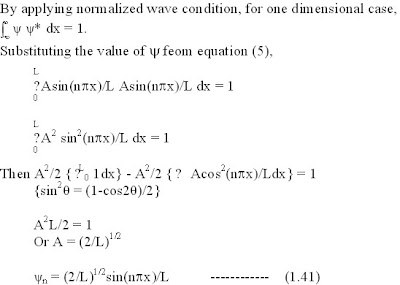
4) The wavefunction for the motion of particles in one dimensional potential box of length ‘a’ is given by yn = D sin npx/a where ‘D’ is normalization constant. The value of ‘D’ is
(04 Marks)
b. Set up time independent one dimensional Schrödinger wave equation. (06 Marks)
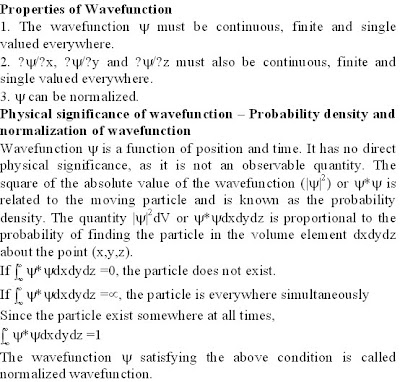
c. Write the physical significance of wavefunction. (04 Marks)
d. A quantum particle confined to one dimensional box of width ‘a’ is in its first excited state. What is the probability of finding the particle over an interval of (a/2) marked symmetrically at the centre of the box? (06 Marks)
June-July 2009
2 a i) According to Max Born approximation, y2 represents
A) Energy density B) Particle density C) Probability density D) Charge density
ii) An electron has a speed of 100 m/s, accurate to 0.005%. The uncertainty in its position is
A) 0.01 m B) 0.0115 m C) 0.024 m D) 0.04 m
iii) An electron is moving in a box of length ‘a’. If ‘y1’ is the wavefunction at x = a/4 with n = 1 and y2 at x = a for n = 2, then y2/y1 is
iv) The lowest quantized energy of a particle of mass ‘m’ in a box of length ‘L’ is given by
A) Zero B) h2/8mL2 C) 2h2/8mL2 D) h2/2mL2 (04 Marks)
b. Explain Heisenberg’s uncertainty principle. Give its physical significance. (06 Marks)
c. Set up time independent one dimensional Schrödinger wave equation. (06 Marks)
d. A quantum particle confined to one dimensional box of width ‘a’ is in its first excited state. What is the probability of finding the particle over an interval of (a/2) marked symmetrically at the centre of the box? (04 Marks)
Dec 09/ Jan 10
2 a i) The normalization of wavefunction is always possible, if
A) y y* dx = infinite B) y y* dx = finite
C) y y* dx =0 D) All of those
ii) Schrödinger’s wave equation is applicable for the particles with
A) Constant energy B) Variable energy C) Only constant potential energy
D) All of these
iii) The ground state energy of an electron in an infinite well is 5.6 meV. If the width of the well is doubled, the ground state energy is
A) 9.92×10-23 J B) 4.48× 10-22 J C) 2.24×10-22 J D) None of these
iv) The wavefunction is acceptable if it is
A) Finite everywhere B) Continuous everywhere C) Single valued everywhere
D) All of these (04 Marks)
b. State Heisenberg’s uncertainty principle and discuss its physical significance.
(06 Marks)
c. Solve the Scrodinger’s wave equation for allowed energy values in case of a particle in a potential box. (10 Marks)
May/June 2010
2 a i) If free electron exists in a nucleus, its energy value must have a minimum energy of about
A) 4 MeV B) 20 MeV C) 20 KeV D) 10 KeV
ii) According to Max Born approximation, y2 represents
A) Energy density B) Particle density C) Probability density D) Charge density
iii) If E1 is the energy of the lowest state of a one dimensional potential box of length ‘a’ and E2 is the energy of the lowest state when the length of the box is halved, then
A) E2 = E1 B) E2 = 2E2 C) E2 = E1/2 D) E2 = 4E1
iv) ) The wavefunction for the motion of particles in one dimensional potential box of length ‘a’ is given by yn = A sin npx/a where ‘A’ is normalization constant. The value of ‘A’ is
(04 Marks)
b. State and explain Heisenberg’s uncertainty principle and prove that nuclei do not contain electron. (08 marks)
c. Discuss the wavefunctions and probability density for particle in an infinite potential well for first two states. (04 Marks)
d. An electron is bound in one dimensional potential well of width 0.18 nm. Find the energy value in eV of the second excited state. (04 Marks)
January 2011
2 a. Choose your answers for the following:
i) The uncertainty in the determination of position of an electron is h/3p. Then, the uncertainty in the determination of its momentum is
A) ¾ B) ¼ C) 4/3 D) 3
ii) The probability of locating a particle is maximum
A) at the centre of the wavepacket B) at the nodes of the wavepacket
C) cannot be determined D) none of these
iii) In Davisson and Germer experiment, when 54 volts was applied to electrons, the pronounced scattering direction was found to be at
A) 900 B) 1200 C) 500 D) none of these
iv) The ground state energy of an electron in an one dimensional infinite potential well of width 2Å is 16 eV. Its energy in third excited state is
A) 32 eV B) 64 eV C) 144 eV D) 256 eV (04 Marks)
b. State and explain Heisenberg’s uncertainty principle (04 Marks)
c. Find the eigen value and eigen functions for an electron in one dimensional potential well of infinite height. (08 Marks)
d. Estimate the time spent by an atom in the excited state during the excitation and de-excitation processes, when a spectral line of wavelength 546 nm and width 10-14 m is emitted. (04 marks)
June/July 2011
A) ![]() B)
B) ![]() C) 0 D)
C) 0 D) ![]()
ii) For a particle in an infinite potential well in its 1st excited state, the probability of finding the particle at the center of box is
A) 0 B) 0.25 C) 0.5 D) 0.1
iii) To become a nuclear constituent, the K.R of e- must be of the order of
A) 20MeV B) 2MeV C) 20eV D) Zero
iv) An electron has speed of 100ms-1 accurate to 0.05%. The uncertainty in its position is
A) 0.01m B) 0.0115m C) 0.024m D) 0.04m (04 Marks)
b. What is a wave function? Explain the properties of a wave function. (04 Marks)
c. Derive the expression for energy Eigen value for an electron in potential well of infinite depth. (06 Marks)
d. A quantum particle confined to one dimensional box of width ‘a’ is in its first excited state. What is the probability of finding the particle over an interval of (![]() marked symmetrically at the centre of box. (06 Marks)
marked symmetrically at the centre of box. (06 Marks)










2 comments:
Why this unit is blank.It is an important unit
Good
Post a Comment